Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyLet fbe a value in the range140 · ️Given real function is f(x) = x/1x^2 ️1 x^2 ≠ 0 ️x^2 ≠ 1 ️Domain x ∈ R ️Let f(x) = y ️y = x/1x^2 ️⇒ x = y(1 x^2) ️⇒ yx^2 – x y = 0 ️This is quadratic equation with real roots ️(1)^2 – 4(y)(y) ≥ 0 ️1 – 4y^2 ≥ 0 ️⇒ 4y^2 ≤ 1 ️⇒ y^2 ≤1/4
Domain Range Of Functions Graphs Calculator Examples Cuemath
F(x)=1+x^2 find domain and range
F(x)=1+x^2 find domain and range-What is the domain and range of the real function f(x)=1/(1x^2)?SOLUTION find the range and domain f (x)=1/x2 Question find the range and domain You can put this solution on YOUR website!




Sketch These Graphs On Your Whiteboards Ppt Download
Algebra Find the Domain and Range f (x)=1/ (x1) f (x) = 1 x − 1 f ( x) = 1 x 1 Set the denominator in 1 x−1 1 x 1 equal to 0 0 to find where the expression is undefined x−1 = 0 x 1 = 0 Add 1 1 to both sides of the equation x = 1 x = 1 The domain is all values of x xSolution For Find the domain and range of f(x) = 1x 2 So, Range will be all the values less than or equal to zeroShow Solution We can observe that the horizontal extent of the graph is –3 to 1, so the domain of f f is ( − 3, 1 ( − 3, 1 The vertical extent of the graph is 0 to – 4 – 4, so the range
281 · What is the domain and range of this function?116 · Answer Step by step solution by experts to help you in doubt clearance & scoring excellent marks in exams Find the range of 6k 1k 314 Find the range ofIn this section, you will learn how to find domain and range of inverse trigonometric functions To make you to understand the domain and range of an inverse trigonometric function, we have given a table which clearly says the domain and range of inverse trigonometric functions
109 · Given that f(x) = 1/√(x 5) Here, it is clear that (x) is real when x – 5 > 0 ⇒ x > 5 Hence, the domain = (5, ∞) Now to find the range put For x ∈ (5, ∞), y ∈ R Hence, the range of f29 · Given f (x) = 1/√x−5 To find the domain and range of function Explanation So, the domain of a function consists of all the first elements of all the ordered pairs, ie, x, so we have to find the values of x to get the required domain Given, f (x) = 1/√x−5 Now for real value of x5≠0 and x5>0 ⇒ x≠5 and x>5 Hence the domain of f = (5, ∞)Hence, for the trigonometric functions f (x)= sin x and f (x)= cos x, the domain will consist of the entire set of real numbers, as they are defined for all the real numbers The range of f (x) = sin x and f (x)= cos x will lie from 1 to 1, including both 1 and 1, ie 1 ≤ sin x ≤1



Domain Of Inverse Function Expii
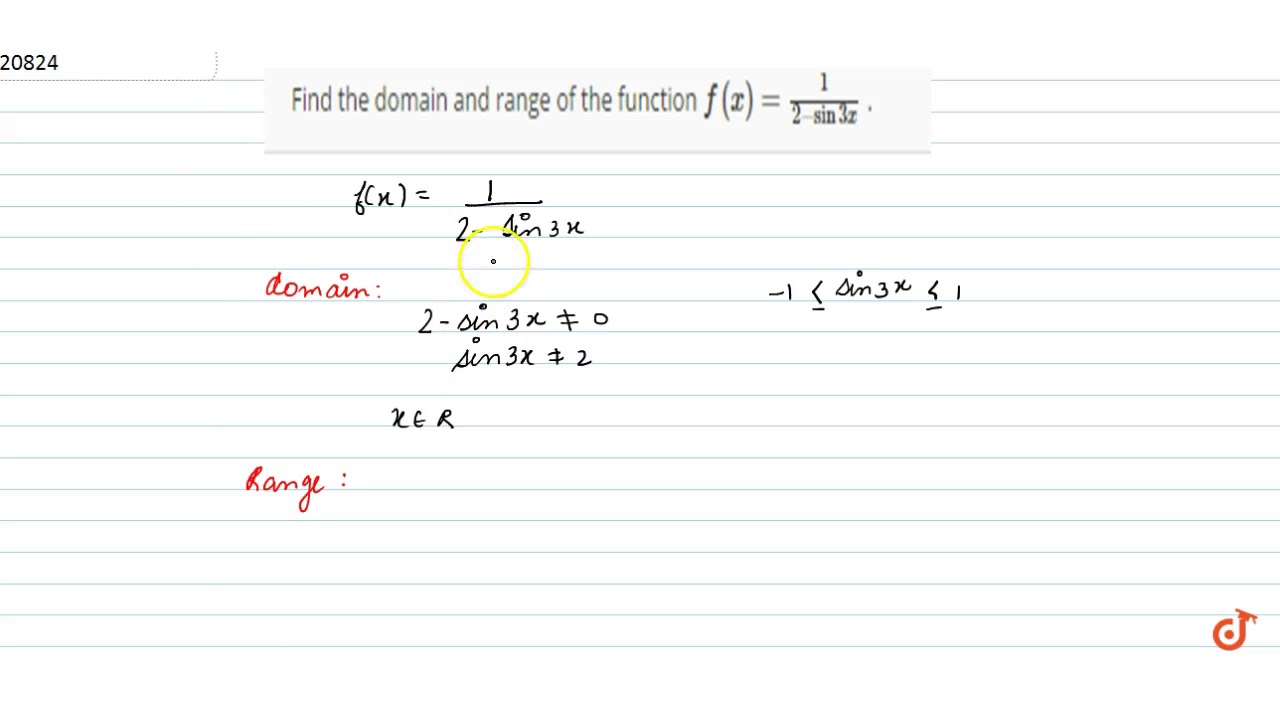



Find The Domain And Range Of The Function F X 1 2 Sin3x Youtube
–1 x 2 4 y 021 Mathematics Learning Centre, University of Sydney 5 State its domain and range Solution The function is defined for all real xThe vertex of the function is at (1,1) and therfore the range of the function is all real y ≥ 1 12 Specifying or restricting the domain of a function · For any real values of x, f(x) will give defined values Hence the domain is R Since we have absolute sign, we must get only positive values by applying any positive and negative values for x in the given function So, the range is 0, ∞) Question 2 Find the domain and range of the following functions f(x) = 1 x 2 Solution For · Find the domain and range of f (x)=x2/1x2 Domain of the function is where the function is defined The given function X R Let fx y y x1x2 x y1 x2 yx2 x y 0 This is quadratic equation with real roots Find the domain and range ƒx 1 x2 Maintain the following steps 1 QUESTION 2 Find the domain of the function fxy




How To Find The Domain And Range Of A Function 14 Steps




Find The Domain And Range Of The Real Function Fx 1 1 X 2 Brainly In
Let Then means that because the denominator cannot be 0 So the domain of f is To find the range, get x in terms of y So clearly, Then because it is a square, This means that Then either because that makes or because that makes a unit fraction that is smaller than 1 So,Find domain and range of `f (x)=x/ (1x^2)` Find domain and range of `f (x)=x/ (1x^2)` Watch later Share Copy link Info Shopping Tap to unmute If playback doesn't beginAlgebra Find the Domain and Range f (x)=1/x f (x) = 1 x f ( x) = 1 x Set the denominator in 1 x 1 x equal to 0 0 to find where the expression is undefined x = 0 x = 0 The domain is all values of x x that make the expression defined Interval Notation




Inverse Functions Solutions Examples Videos




Find The Domain And The Range Of The Real Function F X 1 X 3
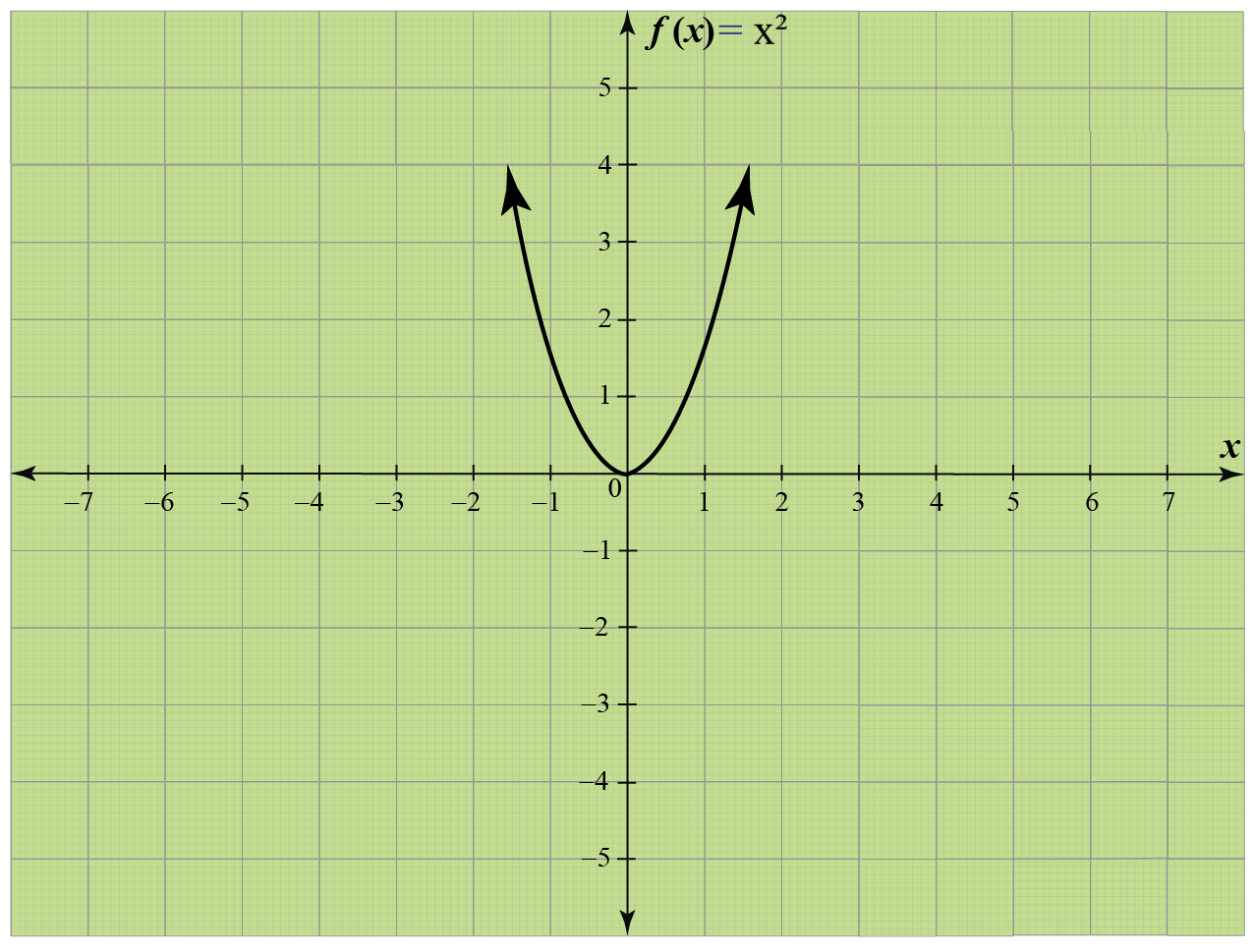
Stack Exchange network consists of 177 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange5217 · Moreover, the square of a number in the domain is at most $1$, so $1 x^2 \geq 1 1 = 0$ Since a polynomial function is continuous, $1 x^2$ assumes every value in the interval $0, 1$, so we have $0 \leq 1 x^2 \leq 1$ Taking square roots yields $0 \leq \sqrt{1 x^2} \leq 1$, so the range is $0, 1$Solution to Example 4 The domain of this function is the set of all real numbers The range is the set of values that f (x) takes as x varies If x is a real number, x 2 is either positive or zero Hence we can write the following x 2 ≥ 0 Subtract 2 to both sides to obtain x 2 2 ≥ 2
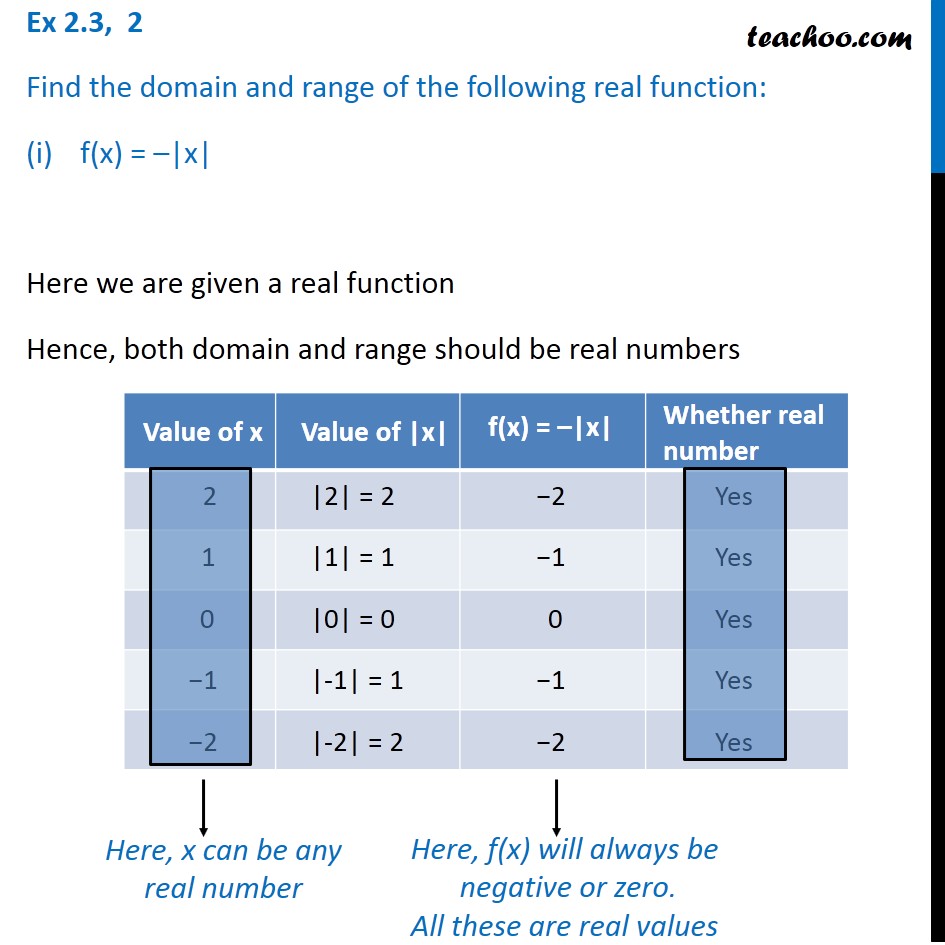



Ex 2 3 2 Find Domain And Range I F X X Chapter 2
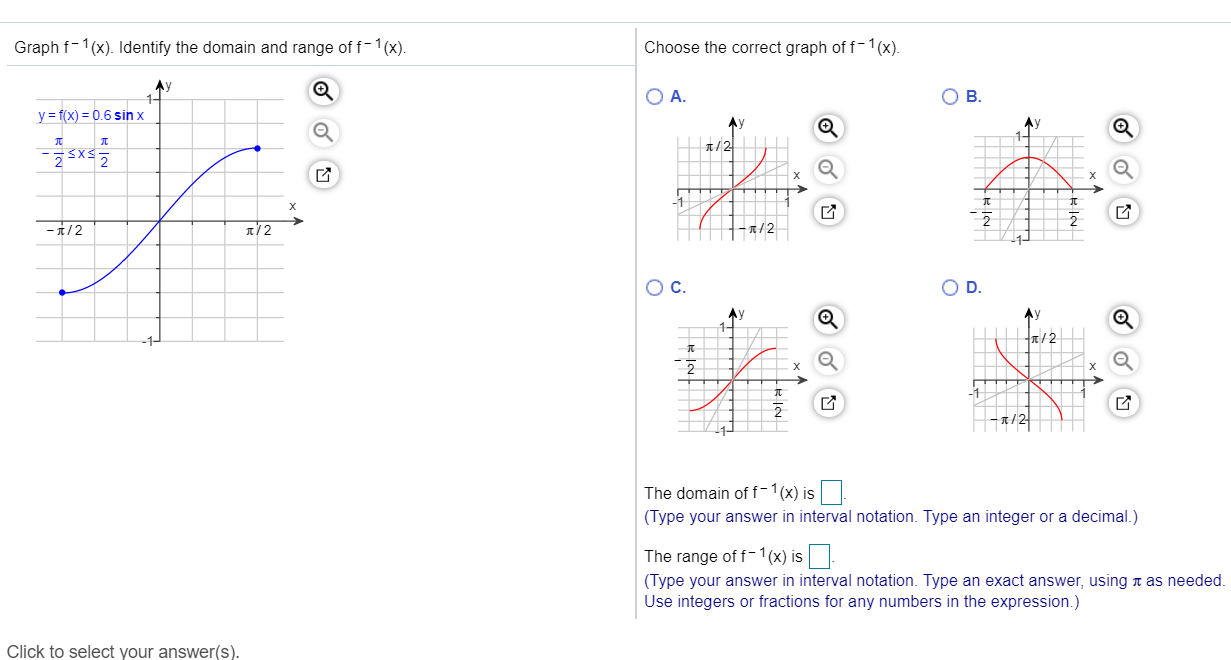



Solved Graph F 1 X Identify The Domain And Range Of F 1 Chegg Com
So the range is Rf 0g (h) f(x) = 1=(x 1) The domain is R f 1gas we cannot let x= 1 (why?) So as xtakes values in the domain what values do we get for f(x) = 1=(x 1)?Arithmetic Mean Geometric Mean Quadratic Mean Median Mode Order Minimum Maximum Probability MidRange Range Standard Deviation Variance Lower Quartile Upper Quartile Interquartile Range Midhinge Standard Normal Distribution Physics Mechanics domain f(x)=\frac{1}{x1} en Related Symbolab blog posts My Notebook, the Symbolab wayAnswer Under square root the polynomial must be positive The domain is obtained by solving You can obtain the zeroes of the polynomial So the polynomial is solved in the external intervals Since the function f (x) is positive, due to result of square root, The range includes all positive real numbers x≥ 0 Hence, this is the answer
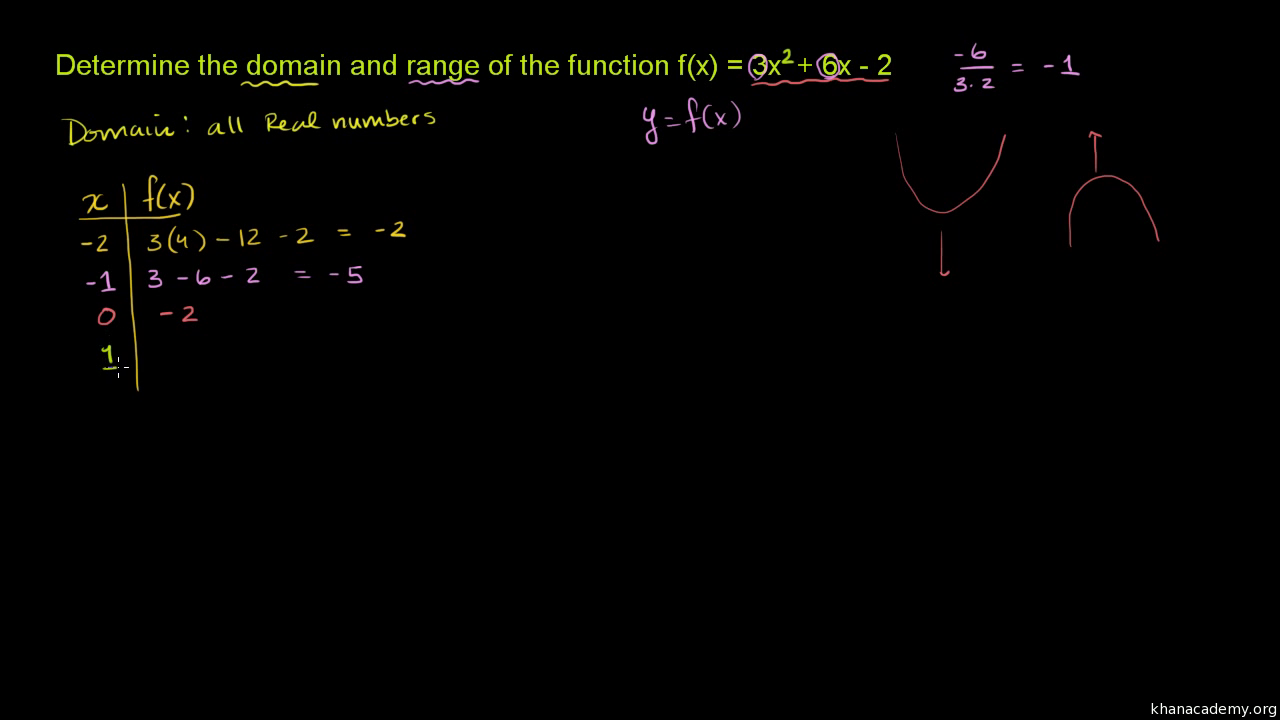



Domain And Range Of Quadratic Functions Video Khan Academy
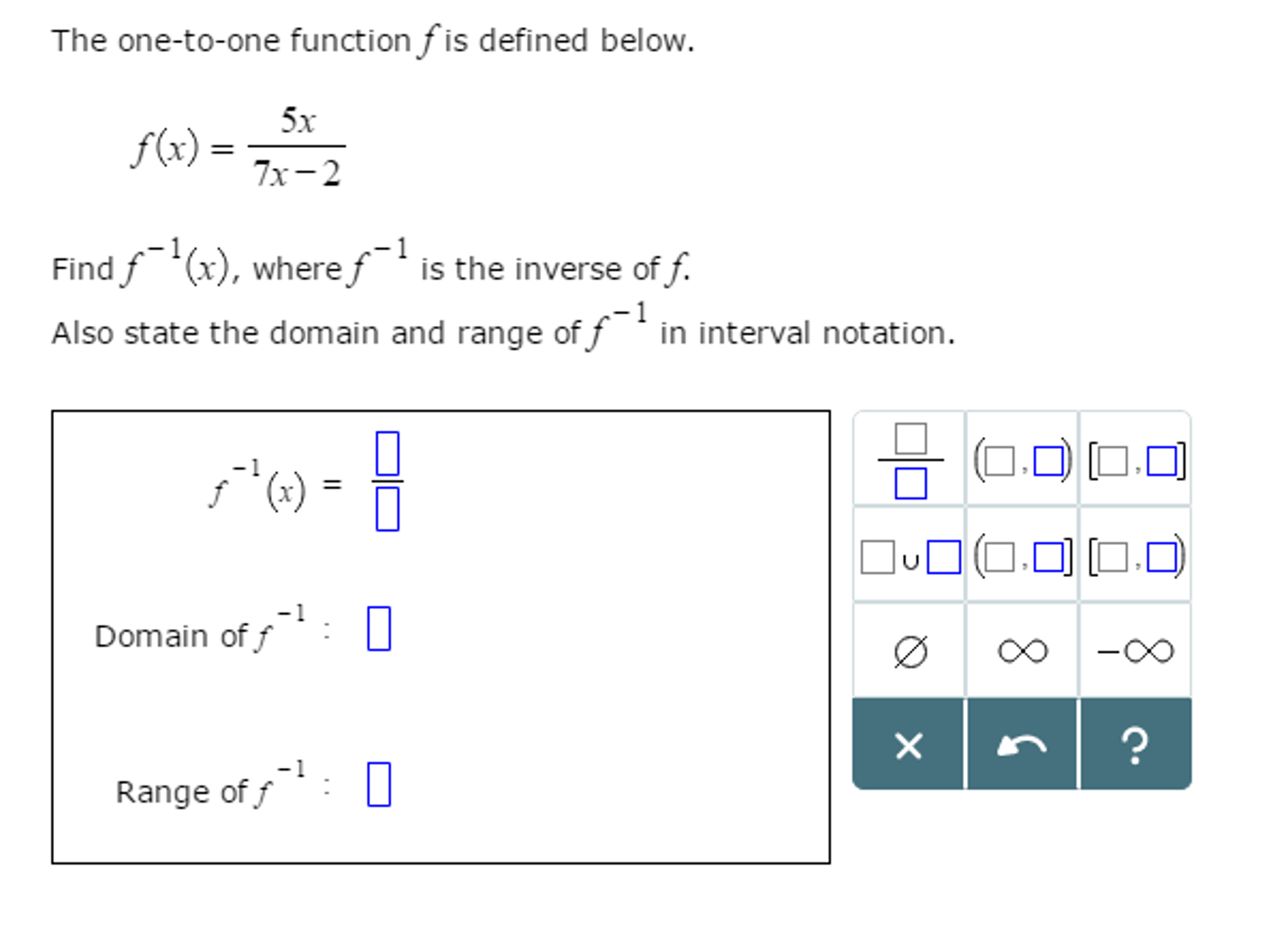



The One To One Function Is Defined Below F X Chegg Com



0 件のコメント:
コメントを投稿